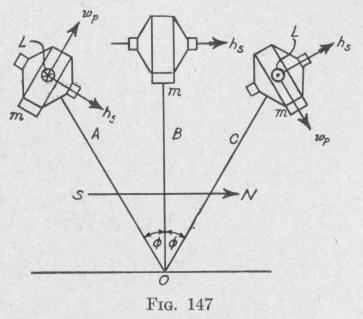
192 NAVIGATIONAL COMPASSES
west, perpendicular to the plane of the diagram - the bow of the ship pointing away from the reader. Suppose that the ship oscillates back and forth about a horizontal axis perpendicular to the plane of the diagram through 0. At the end of a swing, the inertia of the pendulous mass m carries the mass beyond the equilibrium position, thereby developing a torque on the gyrowheel about an axis perpendicular to the plane of the diagram indicated by the sign (*) or (•) marked on the diagram. If the direction of the spin velocity is that represented by the arrow
marked hs, then, when the compass has reached the end of a swing
at A, the north-seeking end of the spin-axle will be deflected away from the reader toward the west. When the gyro-compass comes to the other end of an oscillation at C, the north seeking end of the spin-axle will be deflected toward the east. A succession of back and forth swings of the ship about a horizontal axis produces a succession of vibrations of the gyro-axle but no permanent deflection. Thus, when the ship is pointing east or west, rolling produces a, vibration of the gyro-axle but no fixed deflection from the meridian. Similarly, when the ship is pointing north or south, pitching produces a vibration but no fixed deflection of the gyrocompass. The period of vibration of the spin-axle is that of the oscillation of the ship. As this is never greater than about 15 seconds whereas the period of the entire compass is several minutes, there is no resonant vibration of the spin-axle. Consequently, the amplitude of vibration of the spin-axle due to rolling and pitching is small.
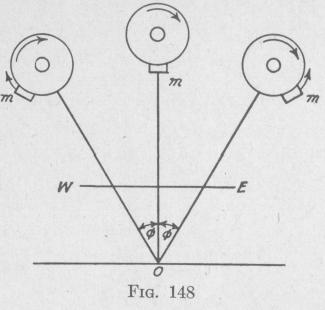
Suppose that the pendulous gyro-compass is on board a ship that
NATURAL ERRORS 191
is either rolling or pitching about an axis through 0 parallel to th spin-axle and perpendicular to the plane of Fig. 148. While th ship oscillates back and forth through the equilibrium position, th
pendulous mass m moves
back and forth with the same period. When the pendulous mass comes to the end of its swing, its inertia carries it beyond its equilibrium position thereby rotating the gyro-casing in the direction indicated by the curved arrow drawn from m. Since this rotation is about an axis parallel to the spin-axle it produces
zero effect on the direction of the spin-axle. Thus, when a ship is on either a north or a south course, rolling produces no deflection of the gyro-compass. When on an east or west course, pitching
produces no deflection.
In the preceding paragraphs, it has been shown that when a ship is steaming on a cardinal course, north, east, south, or west,
neither rolling nor pitching
produces a deviation of the
gyro-compass from the me
ridian. Now, we shall con
sider the effect on the direc
tion of the spin-axle of a
pendulous gyro-compass pro
duced by rolling of the ship
when steaming on an inter
cardinal course. To fix the
ideas, suppose that the course
is NW-SE as indicated in
Fig. 149. The rolling of the
ship causes the compass to
move back and forth along
a path AB, perpendicular to the course, and causes the pendulous
mass to move back and forth relative to the true vertical through
the center of the gyro. After a roll to the north-east, the center
of mass of the pendulous mass will be at a point c east of the true