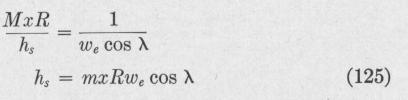188   NAVIGATIONAL COMPASSES

sensitive element will be acted upon by a torque as above. If, however, the course be perpendicular to the spin-axle, the torque will be zero. Representing the meridian component of the mean acceleration of the ship during the short time t by a, there will act upon the gyro a torque L which produces a precessional velocity about the vertical axis of the magnitude,

In this short time t the meridian component of the velocity of the ship and of the compass changes by an amount at. During this time, the gyro-axle turns through the angle

Where $ is the " ballistic deflection " and (v1- vo) is the change in the meridian component of the velocity of the ship in time t.

When the meridian component of the velocity of the ship changes from vo to v,, the resting position of the gyro-axle is de

flected through an angle (o1 - S,), from

the position S,, Fig. 144. Now Q depends

only upon the constants of the compass

and the linear acceleration of the ship,

whereas the meridian-steaming errors de

pend upon latitude and velocity. The

ballistic deflection p and the deflections S, and E,' due to changes in the meridian-steaming error are in the same direction. Consequently, a gyro-compass can be designed with such a period To that, when the instrument is at some particular latitude, the ballistic deflection shall equal the change in the meridiar-steaming error (61' - S,) produced by the change in the velocity of the ship.

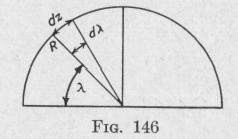
If the ship, starting from rest, moves along a meridian through a distance dz in time dt, with constant linear acceleration a, the velocity at the end of time t will be

During the motion, the latitude of the ship has changed by the amount, Fig. 146:

NATURAL ERRORS 189 where R is the radius of the earth. The rate of change of latitude

is

where dt [= at] is the meridian velocity v' of the ship at the end of

time t. Substituting in (121) the value of at from (122), we obtain - +hn hglligfin rleflentinn

Before equating this value of the ballistic deflection and the value of the meridian-steaming error, we shall express the latter in terms of the time rate of change of latitude. Since the meridiansteaming error S, is so small that we may neglect the difference between S, and tan 5i, (118) may be written

where the meridian component vs cos 0 of the velocity of the ship is represented by v' and the symbol pwe is abbreviated to we.

On substituting in this equation the value of v' from (122), we have for the meridian-steaming error

Now equating the ballistic deflection (123) and the meridiansteaming error (124),

or

When the angular momentum has the value given by this equation, the spin-axle will move without oscillation to the resting position proper to the particular latitude and final velocity. On substituting this value in (88) we find that the period of the azimuthal vibration back and forth through the meridian of the undamped compass that produces zero ballistic deflection error has the value