18   PRINCIPLES OF ELEMENTARY DYNAMICS

to the same axis. Thus, in symbols, the angular momentum with respect to an axis through a point c

h, = K,wC

If a rigid body rotates with an angular acceleration a, about an axis fixed in space, it must be acted upon by a torque (19):

L = Ka

where K is the moment of inertia of the body with respect to the axis of torque. Since angular acceleration is the rate of change of angular velocity, we may write,

L [= Ka] = Kdtw   (28)

The numerator of the right-hand member of this equation is the change of angular momentum. The right-hand member is the time-rate of change of angular momentum. The above equation shows that:

(a) The resultant torque acting on a rigid body about an axis fixed in space, equals the time-rate of change of the angular momentum of the body relative to the given axis.

(b) There can be no increase or diminution of the angular momentum of a body about an axis in space without the action of a corresponding external torque about that axis. This theorem is the analogue in rotation to Newton's First Law of Motion.

(c) When a torque acts upon a body, it produces an angular acceleration, and a rate of change of the angular momentum of the body, about the axis of torque, proportional to the torque.

(d) When a torque acts upon a body, there acts upon some other body another torque of the same magnitude in the opposite direction about the same axis. This theorem is the analogue in rotation of Newton's Third Law of Motion.

(e) When the angular momentum of a body is constant about any axis, then the resultant torque about that axis is zero.

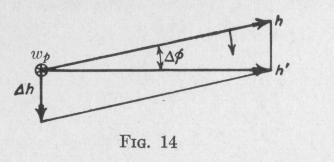
Suppose that at a certain instant a body is spinning with constant angular speed about an axis in the direction of the line h, Fig. 14. The arrow-head on this line indicates the direction of spin about the spin-axis, and the length of the line is proportional to the product of the angular speed and the moment of inertia of the body about the spin-axis. This line represents completely the angular momentum of the body about the spin-axis at the

TRANSLATION AND ROTATION

chosen instant. Suppose that during a short time interval At afterward the direction of the spin-axis changes by a small angle A0 and the magnitude of the angular momentum remains constant. In Fig. 14, h' represents the angular momentum at the end of this

brief time interval, and Ah represents the change of angular momentum during this interval. The rate of change of angular momentum about the spin-axis is

at. This is the value of the

torque about the axis Ah that is required to change the direction of the angular momentum from h to h'. This torque produces a rotation wp about an axis perpendicular to the plane of the torqueaxis and the spin-axis. Thus, a torque acting upon a spinning body about an axis perpendicular to the spin-axis causes the spinaxis to rotate about another axis perpendicular to the plane of the axes of spin and of torque. This is analogous to the case, in translation, in which a force of constant magnitude, acting perpendicular to the direction of motion of a body of constant linear speed, causes the body to describe a circular path.

Experiment. Stand on a rotatable table and hold above the head a bicycle wheel with axle vertical. The wheel should be provided with a rim consisting of 20 to 25 pounds of iron wire. Set the wheel into rotation by pressing against the spokes with a pointed stick. Note that while applying a torque to the wheel, the rotatable table turns in the opposite direction.

Consider a rod suspended at the center of mass by a string. Let the axis of the rod make an angle 0 to the vertical. Suppose that a blow be imparted to one end of the rod in a direction perpendicular to the plane of the string and the axis of the rod. At any instant there is an angular momentum h about an axis perpendicular to the rod and in the plane containing the string and the axis of the rod. The angle 0 is changing and h is changing both in magnitude and in direction. If a force couple of the proper constant magnitude be applied about an axis which is always perpendicular to the plane of h and the axis of the rod, then 0 will remain of constant magnitude. The rod now is rotating with constant angular velocity about the string as axis, tracing the surfaces of two cones having a common apex at the center of gravity of the rod. This applied couple constitutes a centripetal